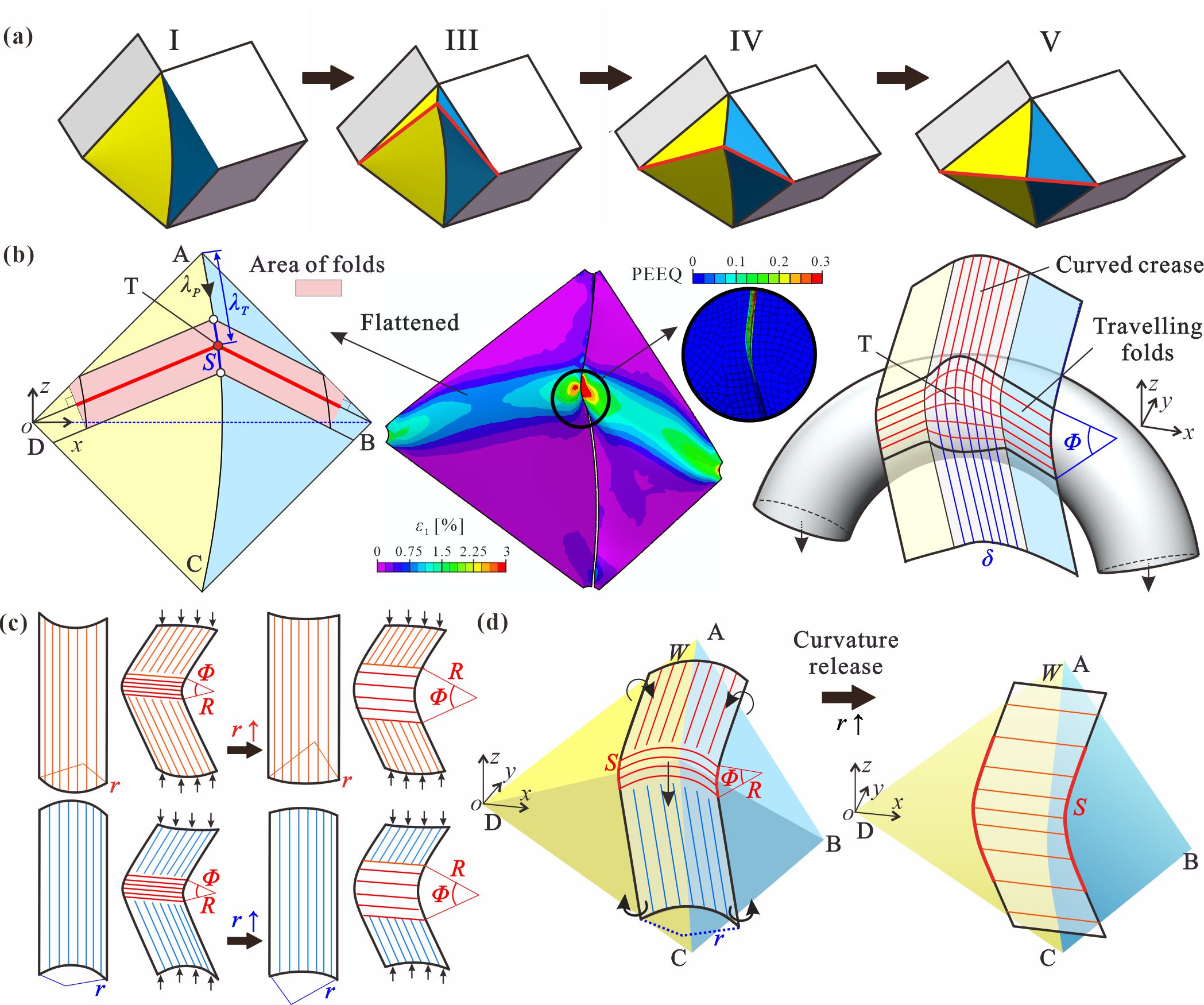
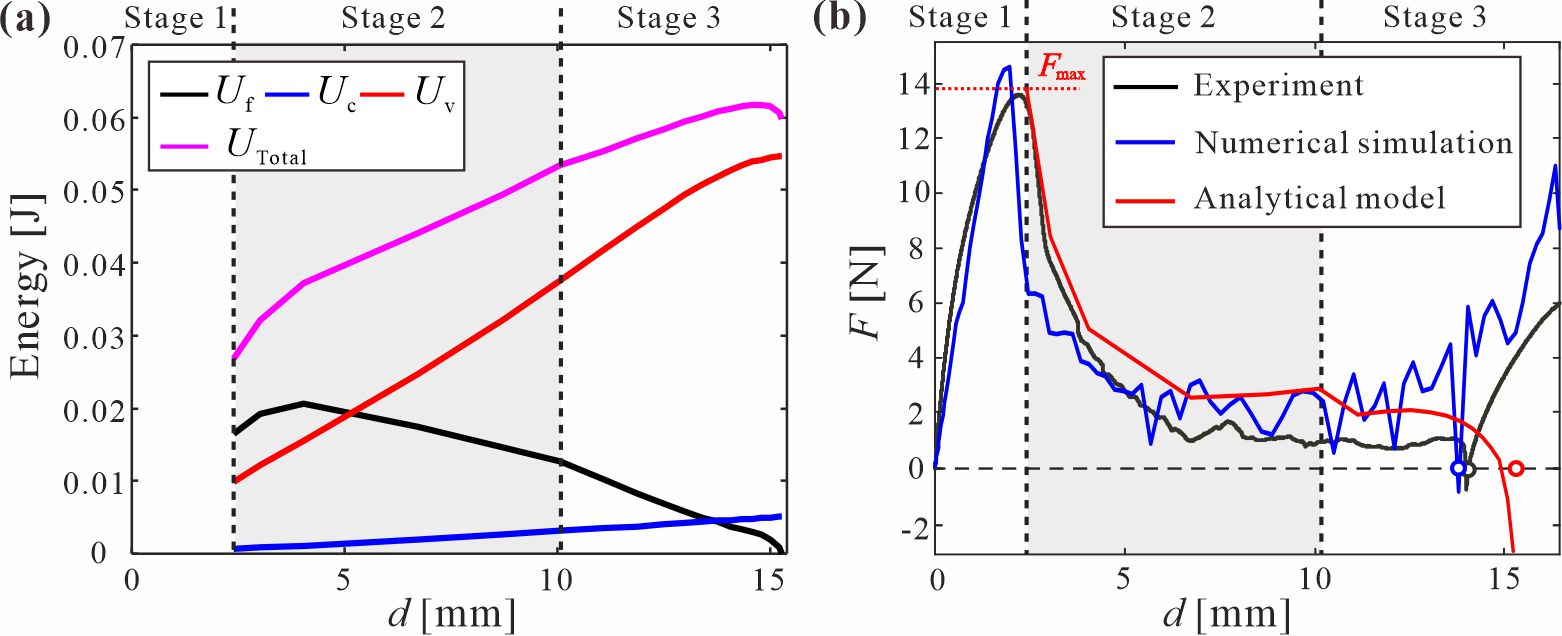
Multi-stable structures capable of rapid switching among different stable states have seen applications in various fields such as energy absorption, robotics, mechanical computing and storage. As a typical form of non-rigid origami, curved-crease origami involves simultaneous rotation of creases and deformation of facets during the folding process, offering a new perspective for the design of multi-stable structures. However, existing curved-crease origami structures primarily deform under free boundary conditions, causing their deformation process to follow the bending mode of the generators. This results in an energy monotonicity similar to that observed in the curved surface formation process. Consequently, curved-crease origami structures can achieve at most two stable states through the elastic bending, flattening, and reverse bending of the facets.

        To address this issue, a research team led by Professor Jiayao Ma from the School of Mechanical Engineering at Tianjin University has designed a series of curved-crease origami structures composed of both planar and curved facets. Under the boundary constraints of the Sarrus linkages formed by the planar facets, the curved facets no longer follow the bending mode of the generators; instead, they exhibit a deformation mode characterized by elastic traveling folds, which resembles the traveling plastic hinges observed in plastic mechanics. Based on this deformation mechanism, an analytical model for the uniaxial compression of curved-crease origami structures has been established. By transforming specific positions of the elastic folds during the movement into physical creases, programmable multi-stable characteristics have been achieved within a single structure. This achievement is published under the title Programmable Multi-Stability of Curved-Crease Origami Structures with Travelling Folds in the flagship journal of solid mechanics, the Journal of the Mechanics and Physics of Solids. PhD student Sibo Chai from the School of Mechanical Engineering at Tianjin University is the first author of the paper, and Professor Jiayao Ma is the corresponding author. Co-authors include postdoctoral researcher Zhou Hu from the School of Astronautics at the Beijing Institute of Technology, Professor Yan Chen from Tianjin University, and Professor Zhong You from the Department of Engineering Science at the University of Oxford. This research is funded by the National Natural Science Foundation and the Science Exploration Award.
        Initially, a design method was proposed by the research team to guide the formation of curved-crease origami structures through boundary constraints, resulting in a series of structures with various cross-sectional shapes. These structures consist of multiple planar and curved facets (Fig. 1a). The planar facets form a Sarrus linkage, which restricts the distribution of generators for the curved facets in the initial configuration (Fig. 1b). The curved facets are composed of two conical surfaces connected by a curved crease along a spatial curve (Fig. 1c). Through the analysis of folding kinematics, the geometric configuration was established under compatibility conditions between the planar and curved facets. Additionally, independent geometric parameters, such as the number of edges in the cross-sections and the half-cone angles of the conical surfaces, were used to regulate the folding angles, surface equations, and crease curvature distributions (Figs. 1d and e).

Fig.1 Geometric design of curved-crease origami structures

        Through experimental and numerical simulation methods, the mechanical response of travelling folds formed in the curved-crease origami unit under uniaxial compressive loading was investigated. Guided by the Sarrus linkage boundary, the curved facets undergo symmetric elastic buckling deformations, gradually forming a pair of asymmetric elastic folds. The fold manifests as a high local stress strip that connects the vertices of the cone and intersects at the curved crease, exhibiting a bending deformation that approximates a cylindrical surface (Fig. 2). The folds initiate near the top vertex and propagate toward the center of the curved surface as loading progresses, accompanied by a decrease in reaction force, which subsequently flattens (Fig. 2f). As the two traveling folds approach horizontal alignment, they suddenly release the bending curvature, rapidly deforming to reach a second stable state. Based on the deformation of the structure, the process can be divided into three stages: the generation, propagation, and release of the traveling folds.

Fig.2 Deformation process of curved-crease origami structures

        To further establish an analytical model of the travelling folds, an equivalent discrete geometry was constructed to elucidate the deformation mechanism of these folds from the perspective of geometric compatibility (Fig. 3a). On this basis, three deformation mechanisms were introduced: cylindrical bending of the traveling folds, vertex stretching at the intersection of the folds, and equivalent tape spring deformation during the curvature release stage (Figs. 3b-d). Based on these deformation mechanisms, three forms of energy present during the compression process were calculated: elastic strain energy from the bending of the traveling folds, elastic strain energy from crease folding, and plastic energy from vertex stretching (Fig. 4a). The results from the analytical model characterized the overall trend of the force-displacement curve and predicted the initial peak force as well as the position of the second stable state under loading (Fig. 4b).

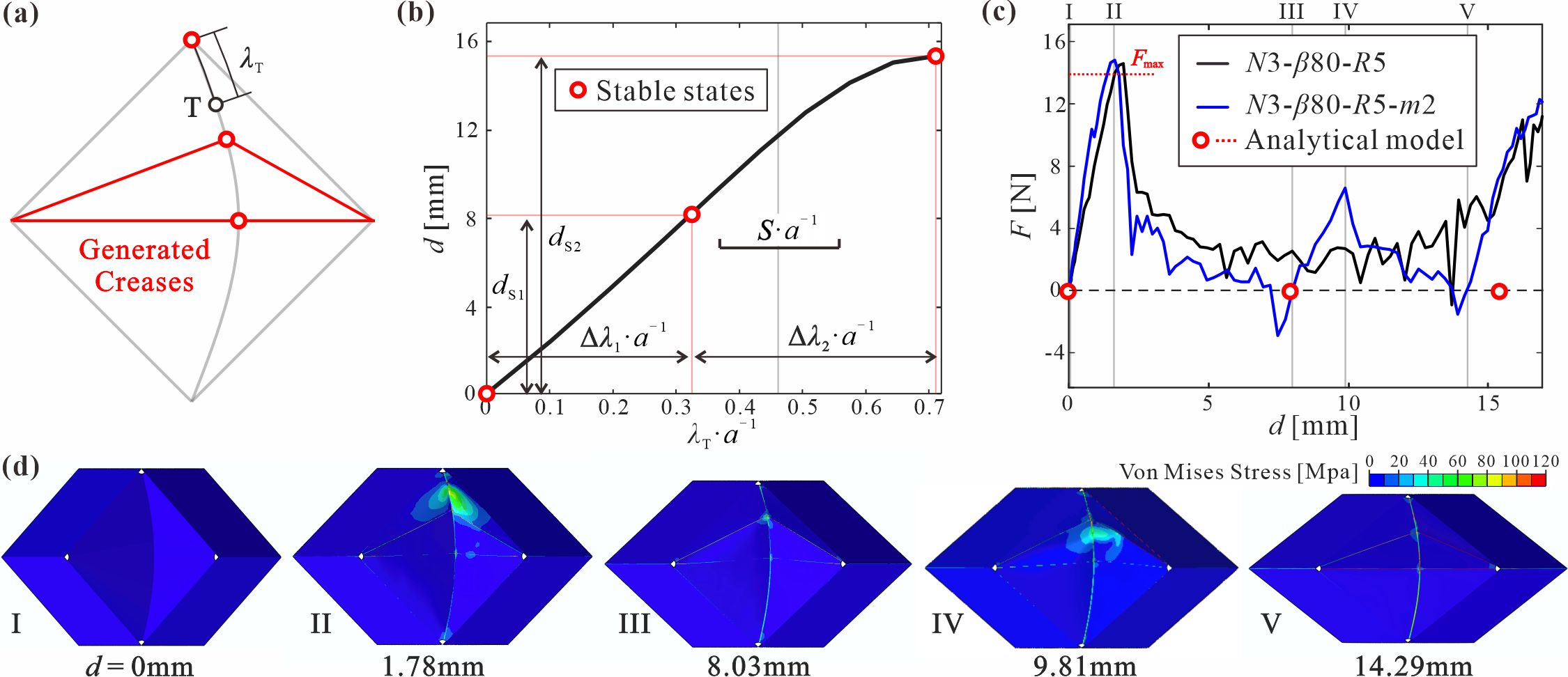
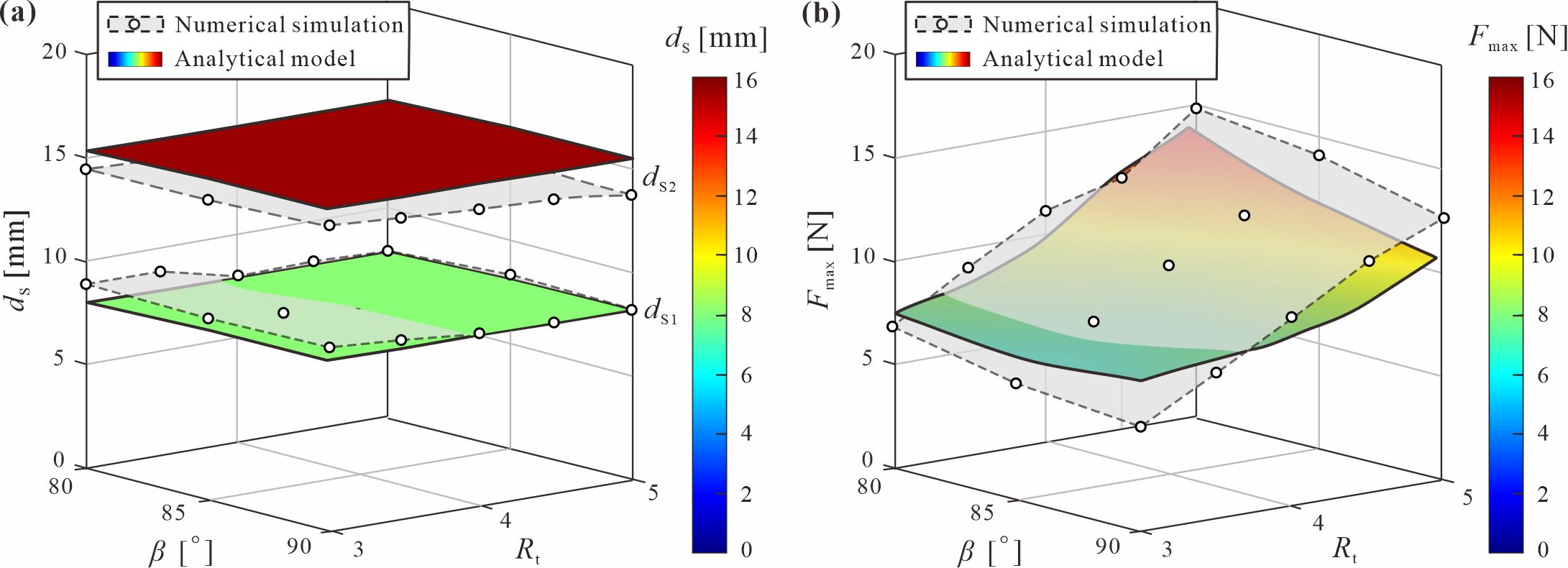
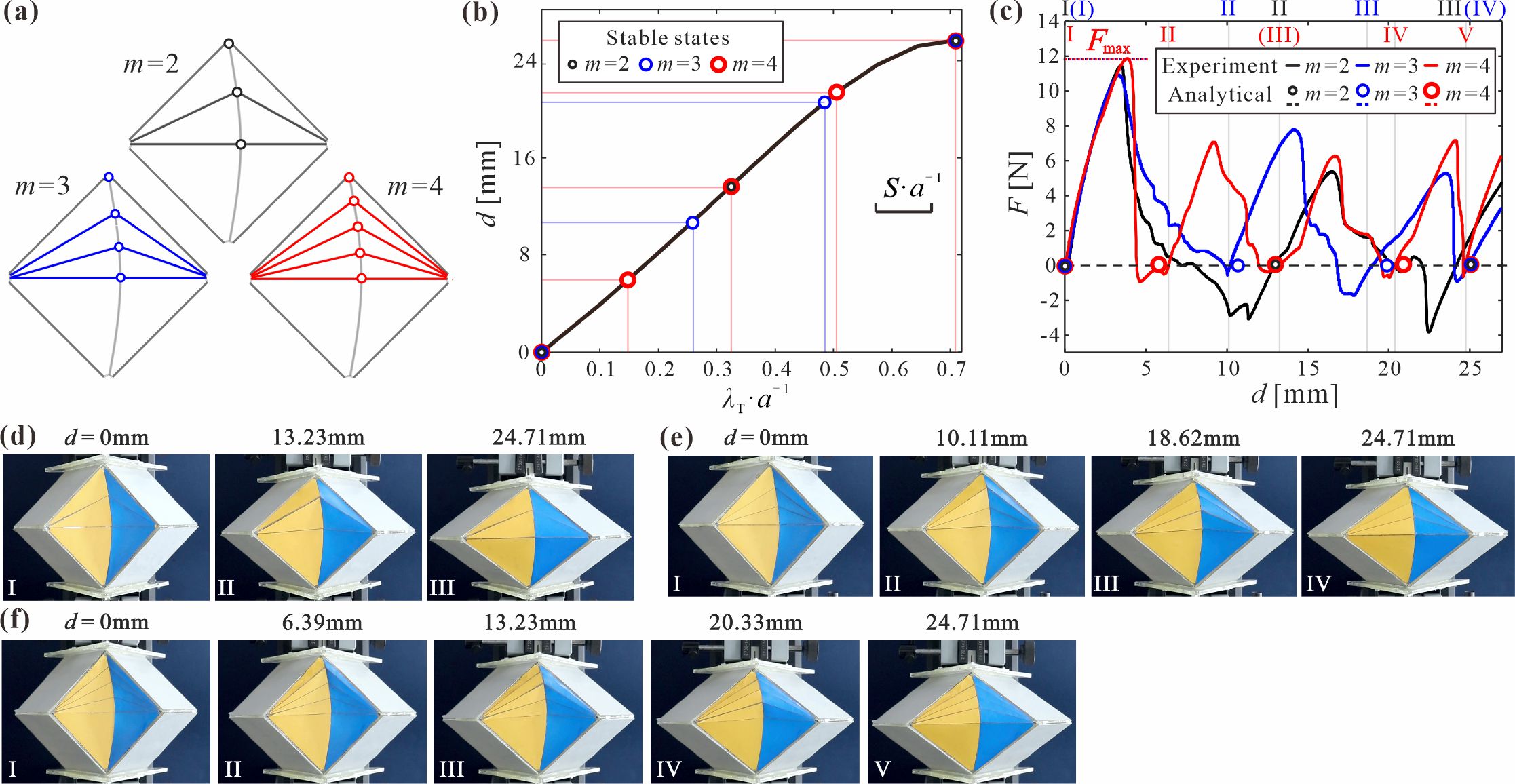
Fig.3 Mechanism modeling of travelling folds, vertex stretching and equivalent tape spring

Fig.4 Analytical results of energy and force

        According to the deformation mechanism of traveling folds, if the generators swept by the folds during their movement are transformed into physical creases, the strain energy of the structure will significantly decrease when the folds reach these creases, thereby creating additional stable states. Based on this crease transformation method, a tri-stable unit with two transformation points was designed (Fig. 5a), allowing for the programming of the number and loading distance of stable states according to the quantity and position of the crease transformations (Figs. 5b and 5c). Furthermore, the introduction of new creases does not affect the initial buckling deformation (Fig. 5d), enabling the analytical model to accurately predict the initial peak force of the multi-stable structure.

Fig.5 Crease transformation method and construction of multi-stability

        Further investigation was conducted on the impact of crease curvature parameters and the thickness ratio of facets to creases on the distances between adjacent stable states and initial peak forces. The analytical model and numerical simulation results indicate that the positions of stable states are almost independent of the design parameters. However, increasing the initial curvature of the creases or the thickness ratio significantly enhances the initial peak force (Fig. 6). In contrast to existing studies on multi-stable units, which can achieve programmability in at most two variables, such as the number of stable states and initial peak forces, or the positions of stable states and energy barriers, the curved crease unit proposed in this study enables full programming capabilities of both the number and positions of stable states, as well as the initial peak force. This full programmability was further validated through compression experiments on curved-crease origami units designed to have three, four, and five stable states (Fig. 7).

Fig.6 Programable multi-stability based on geometric parameters

Fig.7 Experimental validation of programable multi-stability

        In summary, this study proposes a series of curved-crease origami structures guided by constrained boundaries, showcasing a unique deformation mechanism for the generation, propagation, and release of travelling folds. Based on analytical models and crease transformation methods, the multi-stable characteristics of the curved-crease origami units have been achieved, along with full programming of the number and location of stable states, as well as the initial peak force. This work opens a new pathway for the development of generic multi-stable structures with programmable mechanical properties.

Chai S, Hu Z, Chen Y, You Z, Ma J*. Programmable multi-stability of curved-crease origami structures with travelling folds. Journal of the Mechanics and Physics of Solids, 2024, 193, 105877.
(https://doi.org/10.1016/j.jmps.2024.105877)